Abstract
Let \(X\) be a Banach space and \(Y\) a normed space, and \(P:X\rightarrow Y\) a nonlinear operator. In order to solve the equation \(P\left( x\right)=0\), we consider the iterative method \(x_{n+1}=x_{n}+\varphi \left(x_{n}\right) \), where \(\varphi:X\rightarrow X\). We give some sufficient semilocal conditions relating \(\varphi\) and \(P\) for these iterations to converge to a solution with a given convergence order. As particular instances, we obtain convergence results for the Newton, Chebyshev and Steffensen mehods.
Authors
Ion Păvăloiu
(Tiberiu Popoviciu Institute of Numerical Analysis)
Title
Original title (in French)
Sur les procedées itératifs à un ordre élevé de convergence
English translation of the title
On the iterative methods with high convergence orders
Keywords
iterative methods in normed spaces; convergence order; Newton type method; Chebyshev type method; Steffensen type method; semilocal convergence
Cite this paper as:
I. Păvăloiu, Sur les procedées itérative à un order élevé de convergence, Mathématica, 12(35) (1970) no. 2, pp. 309-324 (in French).
About this paper
Journal
Mathematica
Publisher Name
Academia Republicii S.R.
DOI
Not available yet.
Print ISBN
Not available yet.
Online ISBN
Not available yet.
References
[1] Kantorovici, L. V., Functionalıi analiz i pricladnaia matematica. U.M.N., 28, 3 (1948).
[2] Kantorovici, L. V., O metodı Niutona. Trudı mat. i-ta im. Steklova. 28, pp. 104–144 (1949).
[3] Janko, B, si Goldner, G., Despre rezolvarea ecuatiilor operationale cu metoda lui Cebısev. (II), Studia Univ. Babes-Bolyai Cluj 2, pp. 55–58 (1968).
[4] Ghinea, Monique, Sur la resolution des equations operationnelles dans les espaces de Banach, Revue Francaise de traitement de l’information 8, pp. 3–22 (1965).
[5] Ostrowski, A. M., Resenie uravnenii i sistem uravnenii. Izd. inost. lit., Moskva, (1963).
[6] Pavaloiu, I., Asupra rezolvarii ecuatiilor operationale prin metode de iteratie de ordin superior. Lucrarile colocviului de teoria aproximarii functiilor (rezumat), Cluj 15-20 septembrie 1967.
[7] Pavaloiu, I., Sur la methode de Steffensen pour la resolution des equations operationnelles non lineaires. Revue Roumaine des Mathematiques Pures et Appliquees, XIII, (1968) 6, pp. 857–861.
[8] Pavaloiu, I., Asupra operatorilor iterativi, Studii si Cercetari matematice (in print); appeared as: 23 (1971) no. 10, pp. 1567–1574.
[9] Pavaloiu, I., Interpolation dans des espaces lineaires normes et applications. Mathematica, Cluj, vol. 12 (35), 1, 1970, pp. 149–158.
[10] Stein, M. L., Sufficient conditions for the Banach spaces. Proc. Amer. Math. Soc. 3, pp. 858–863 (1952).
[11] Traub, J, F., Iterative methods for the solution of equations. Prentice-Hall. Inc. Englewood Cliffs N. J. 1964.
Paper (preprint) in HTML form
Sur les procédés itératifs
à un ordre élevé de convergence
Soit un espace de Banach et
| (1) |
une équation opérationnelle où l’opérateur est défini sur l’espace et avec des valeurs dans l’espace linéaire normé .
Pour la résolution de l’équation (1) on consière en général un autre opérateur défini sur l’espace et avec des valeurs dans le même espace.
Définition 1.
On dit que l’opérateur est un opérateur itératif attaché à l’équation (1) si tout élément pour lequel , est un point fixe pour l’opérateur
A l’aide de l’opérateur itératif on attachera à l’équation (1) le procédé itératif suivant.
| (2) |
Définition 2.
Si , l’opérateur itératif et le nombre réel et positif satisfont aux conditions:
a) pour où est un nombre naturel.
b)
Alors on dira que le procédé itératif (2) possède l’ordre de convergence
En ce qui concerne la notion d’ordre de convergence introduite précédemment, a lieu le lemme suivant.
Lemme 1.
Démonstration.
Puisque le procédé (2) possède l’ordre de convergence il en résulte qu’il existe un élément pour lequel
| (3) |
où est une constante réelle et positive pour laquelle est remplie aussi la condition a) de la définition 2.
En employant la condition a) de la définition 2, pour on obtient
d’où, en écreivant l’on déduit
Si on applique cette inégalité successivement pour on obtient
ou, si on tient compte de (3) on a
Puisque il en résulte que
Si et l’opérateur est continu, il en résulte qu’on a l’égalité:
C’est-à-dire
ce qu’il fallait démontrer. ∎
Dans la définition 2 où on a précisé la notion d’ordre de convergence d’un opérateur itératif on n’a pas inclus la condition que la suite donnée par le procédé (2) soit convergente.
En ce sens, on est parti de l’idée qu’en pratique on est assez rarement conduit par certains procédés à la solution exacte des équations de forme (1). D’habitude, pour les besoins de la pratique, on peut se borner à une seule solution approchée. Un des critères simples d’appréciation du fait que est une solution approchée pour l’équation (1) consiste à vérifier dans quelle mesure le nombre réel et positif est proche de zéro. Évidemment, plus il sera proche de zéro, plus on considérera que la solution approchée trouvée peut satisfaire plus exactement aux requêtes du problème pratique.
Dans ce qui suit, dans tous les problèmes de convergence qu’on étudiera, concernant l’équation (1) et le procédé (2) on examinera tant la manière dont la suite converge vers zéro que la manière dont la solution approchée, approche la solution exacte.
On présentera mainenant un critère géneral de convergence pour la suite fournie par le procédé (2). Ce résultat pourra être considéré aussi comme un critère d’existence de la solution pour l’équation (1). Pour cela on supposera que l’oṕrateur itératif a la forme:
| (3′) |
Théorème 2.
[6]. Soit et Si l’élément et le nombre réel peuvent être choisis de telle sorte que dans la sphère soient remplies les conditions suivantes:
a) l’opérateur admet des dérivées (dans le sens de Fréchet) jusqu’à l’ordre inclusivement et pour tout
b) Il existe une constante réelle et non-négative pour laquelle a lieu l’inégalité
pour tout
c) Il existe une constante réelle et positive pour laquelle a lieu l’inégalité
d) où
e)
a’) L’équation (1) a au moins une solution
b’) La suite est convergente et
c’)
d’)
e’)
Démonstration.
De la condition c) il résulte l’inégalité suivante
| (4) |
d’où on déduit l’inegalité suivante:
c’est-à-dire En utilisant les hypothèses a), b) et c) et la formule de Taylor généralisée on en déduit
c’est-à-dire qu’a lieu l’inégalité
Puisque il résulte que pour a lieu l’inégalité c’).
On suppose maintenant que pour tout et on suppose encore que pour les mêmes valeurs de ont lieu les inégalités
Dans ces hypothèses on montrera que et que l’inégalité c’) a lieu aussi pour
Si on procède de la même manière que précédemment, en partant des hypothèses faites on obtiendra les inégalités suivantes.
| (5) |
En multipliant ces inégalités par on déduit
et en écrivant on aura
| (5′) |
ce qui, par application succesive, donne
| (6) |
Des inégalités (6) et de l’hypothèses c) on déduit
d’où résulte la valabilité de la conclusion c’). De (6) résulte aussi la conclusion e’).
On démontrera à présent que En effet on a
d’où il résulte que Pour démontrer la convergence de la suite on montrera que cette suite est fondamentale. En effet pour on a
| (7) |
d’où tenant compte de l’hypothèse d) il résulte que la suite est convergente puisque est un espace complet.
Soit alors en passant à la limite dans l’inégalité (7) pour on a
c’est-à-dire l’inégalité d’). Des inégalités (5′) et de l’hypothèse d) résulte la propriété f’).
Du fait que est dérivable en il résulte qu’il est un opérateur continu et donc en passant à la limite dans les inégalités (6) on déduit facilement que c’est-à-dire c’est-à-dire la propriété a’). ∎
Remarque.
Dans la démonstration du théorème 2 on n’a pas employé le fait que les conditions b) et c) sont remplies sur toute la sphère On obtient les mêmes résultats si on suppose que ces conditions sont remplies seulement pour les éléments de la suite fournis par le procédé (2). ∎
On appliquera maintenant le théoréme 2 pour présenter quelques résultats dans le cas de procédés itératifs particuliers.
Le procédé itératif de Newton-Kantorovici est obtenu en employant l’opérateur itératif:
Dans ce cas-là, l’opérateur qui intervient dans le théorème précédent a la forme
En appliquant la théorème 2 pour et on demontre facilement le théorème suivant:
Théorème 3.
111Ce théoréme a été démontré par Misovski. I.P. (Trudî Mat. i Steklova 28, 145-147 (1949).Soit et Si et peuvent être choisis de telle sorte que les conditions suivantes soient satisfaites:
a) L’opérateur existe et il est borné pour c’est-à-dire
b) L’opérateur admet une dérivée seconde (dans le sens de Fréchet) et cette dérivée est bornée, c’est-à-dire, pour tout
c)
d) où .
Alors ont lieu les propriétés suivantes pour l’équation (1) et le procédé de Newton-Kantorovici.
a’) L’équation (1) a au moins une solution
b’) La suite est convergente et
c’)
d’) ou
e’) Le procédé de Newton-Kantorovici possède l’ordre de convergence 2.
Pour cette méthode on considère le procédé itératif suivant:
Soit
| (8) |
et la méthode itérative correspondente est
| (9) |
Si on écrit
alors l’opérateur a la forme (3′).
On vérifie tout de suite l’égalité:
| (10) | |||
Théorème 4.
Soit et Si l’élément et le nombre réel peuvent être choisis de telle sorte que les conditions suivantes soient remplies:
a) l’opérateur admet des dérivées (dans le sens de Fréchet) jusqu’à l’ordre trois inclusivement pour tout et
b) L’opérateur existe et il est borné, c’est-à-dire pour tout
c) Soit
On suppose qu’on a l’inégalité:
Dans ce qui suit, on étudiera la convergence du procédé itératif de Steffensen. Dans le travail [8] on a montré que l’opérateur itératif de Steffensen nous fournit les deux procédés itératifs équivalents suivants:
| (11) |
et
| (12) |
Relativement à ce procédé, il existe le théorème suivant:
Théorème 5.
Soit un élément pour lequel sont remplies les conditions ci-dessous:
a)
b) Les nombres réels et non-négatifs et satisfont à inégalité
est un nombre naturel.
c) Soit un nombre réel et non-négatif.
On écrira où
et On suppose que pour tout la différence divisée admet une inverse bornée par le nombre c’est-à-dire
d) Pour tout les différences divisées et sont symétriques par rapport aux noeuds donnés et on a les inéqalités:
e) L’opérateur itératif possède l’ordre dans la sphère c’est-à-dire qu’on a l’inégalité et l’opérateur est continu en
Si les conditions a)–e) sont remplies alors on a les propriétés suivantes:
a’) Les éléments de la suite fournie par le procédé (11) appartiennent à la sphère .
b’) Les éléments de la suite appartiennent à la sphère
c’) L’équation (1) possède au moins une solution
d’) La suite est convergente et
c’) L’ordre de convergence du procédé (11) est
Démonstration.
Si on écrit et on tient compte du fait qu’on a supposé que la différence divisée est symétrique par rapport aux noeuds, on a
Pour établier l’inégalité précédente on a tenu compte du fait que ce qui résulte de l’inégalité
De la même manière on a
c’est-à-dire
On supposera maintenant qu’on a construit à l’aide du procédé (11) les éléments et On montrera que et En effet on remarque facilement que pour on a
| (13) |
En multipliant la dernière inégalité par et en écrivant on obtient les inégalités:
d’où il résulte
En tenant compte de ces inégalités et de (13) on déduit:
d’où il résulte l’inégalité
c’est-à-dire
et par conséquent
Il en résulte immédiatement l’inégalité:
c’est-à-dire
De ce fait les conclusions a’) et b’) sont démontrées.
On montrera maintenant que la suite est convergente. Pour cela on montrera que cette suite est une suite fondamentale. On a
| (14) |
ce qui nous montre que la suite est une suite fondamentale et comme l’espace est supposé complet il en résulte que cette suite est convergente.
Soit:
En passant à la limite dans l’inégalité (14) pour on a
| (15) |
Cette inégalité nous donne aussi une évaluation de l’erreur après pas d’itération. Puisque on remarque que l’erreur tend rapidement vers zéro lorsque croît.
En passant à la limite dans l’inégalité
et en tenant compte de la continuité de on a on ce qui démontre la conclusion c’). Évidemment, l’inégalité (15) pour nous montre que
La conclusion e’) résulte du fait que l’inégalité (15) a lieu pour tout et de l’hypothèse b).
On présentera maintenant une application numérique des résultats exposés dans cette note. ∎
Application.
On considère l’équation intégrale du type Fredholm
| (1′) |
Cette équation admet, en plus de la solution une autre solution
Pour la résolution de l’équation (1′) on appliquera d’abord la méthode de l’itération simple. Soit donc où est un nombre réel fixé, une solution initiale, et
| (2′) |
Du procédé précédent il résulte que a la forme:
où
| (3′) |
Si le procédé itératif (3′) converge, alors à la limite on obtient évidemment une des solutions de l’équation
| (4′) |
L’éguation (4′) admet les solutions
| (5′) |
et
| (6′) |
Suivant la terminologie adoptée par A.M. Ostrowski [5], on remarque que est un point de ”contraction” pour l’équation (4′) puisque si on choisit alors le procédé itératif (3′) converge et on a D’autre part le point est un point ”répulsif” pour l’équation (4′) puisque la fonction
satisfait à la condition:
En appliquant les résultats du lemme 4.2 [5, p. 36] on peut en déduire la conclusion que le procédé (3′) ne convergera jamais vers la solution (6′) quelque soit le choix de la valeur initiale.
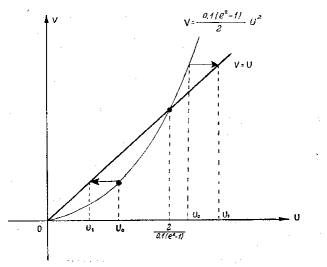
Graphiquement, ce fait se présente ainsi (Fig. 1).
Si on représente graphiquement dans le système de coordonnées les courbes et alors les solutions de l’équation (4′) ne sont autre chose que les abscisses des points d’intersection des deux courbes.
Sur la figure ci-dessus on remarque qu’en choisissant par example on aura c’est-à-dire qu’à l’aide du procédé (3′) on obtient Si alors c’est-à-dire que le procédé (2′) est divergent. En prenant pour le nombre la valeur approchée et en employant le procédé (3′) on obtient les résultats compris dans le tableau 1 222Dans ce tableau on n’a introduit que les valeurs qui suffisent pour en déduire les conclusions nécessaires sur la convergence des suites d’itérations correspondentes.
Tableau 1
| . | |||
| . | |||
| . | |||
| . | |||
| . | |||
| . | |||
Évidemment, les résultats des calculs nous montrent que si on prend alors la suite des approximations successives a comme limite la valeur Si on prend par exemple alors
Pour mieux illustrer la valabilité des conclusions on a pris pour la valeur qui diffère très peu de la solution exacte, mais laquelle est plus grande que celle-ci. Dans ce cas-là, en effectuant la suite des itérations successives on remarque que, même si après les premières quatre itérations la troisième chiffre décimal du résultat est conservé, ce qui pourrait nous donner l’illusion d’une possibilité de convergence du procédé, on sera convaincu pourtant, en continuant le procédé, que même dans ce cas on a
On a montré ici, par plusieurs moyens que la solution de l’équation (1′) ne peut être obtenue que par itération simple. En partant des mêmes approximations initiales on résoudra maintenant l’équation (1′) en appliquant la méthode de Steffensen. Pour cela on remarquera que l’équation (1′) peut être mise sous la forme
où
En prenant, comme dans le procédé précédent, une solution initiale de la forme on trouve et un calcul simple nous montre que la méthode de Steffensen nous donne pour la deuxième approximation l’expression suivante
On en déduit qu’en général, la méthode de Steffensen nous conduit à la suite suivante d’approximations
où
| (7′) |
En prenant pour les valeurs et on obtient les résultats compris dans le tableau 2.
Tableau 2
En analysant ce tableau, il en résulte que si on choisit convenablement l’approximation initiale à l’aide de la méthode de Steffensen on obtient la soltuion
Si dans le procédé (7′) on part d’une approximation initiale assez aprochée de zéro, alors on obtiendra la deuxième solution L’exemple étudié précédemment illustre la valabilité des résultats théoriques obtenus dans cette note dans les sens suivants:
- il a des cas où la méthode de l’itération simple ne converge pas vers la solution cherchée, quelque soit le choix de l’approximation initiale. Mais en appliquant la méthode de Steffensen et en choisissant l’approximation initiale d’une manière convenable on obtiendra la solution cherchée. (Ce fait est valable pour n’importe quelle méthode qui possède un ordre de convergence supérieur ou égal à 2).
-la méthode de Steffensen converge rapidement.
Remarquons, par exemple, qu’en partant de l’approximation intiale la méthode de l’itération simple est divergente, tandis que la méthode de Steffensen nous conduit au résultat désiré en 6 pas.
Pour on va essayer d’illustrer numériquement la rapidité de la convergence de la suite vers zéro.
Tableau 3
Dans ce tableau et sont les valeurs approchées (numériques) des fonctions et de l’opérateur On en déduit qu’en prenant alors, pour tout sont satisfaites les inégalités
Ces inégalités caractérisent l’ordre de convergence de la méthode de Steffensen. Plus précisément elles nous montrent qu’il est égal à deux.
Bibliographie
- [1] Kantorovici, L. V., Funcţionalîi analiz pricladnaia matematica. U.M.N., 28, 3 (1948).
- [2] Kantorovici, L. V., O metodî Niutona. Trudî mat. i-ta im. Steklova. 28, pp. 104–144 (1949).
- [3] Jankó, B, şi Goldner, G., Despre rezolvarea ecuaţiilor operaţionale cu metoda lui Cebîşev. (II), Studia Univ. Babeş-Bolyai Cluj 2, pp. 55–58 (1968).
- [4] Ghinea, Monique, Sur la résolution des équations opérationnelles dans les espaces de Banach, Revue Française de traitement de l’information 8, pp. 3–22 (1965).
- [5] Ostrowski, A. M., Reşenie uravnenii sistem uravnenii. Izd. inost. lit., Moskva, (1963).
- [6] Păvăloiu, I., Asupra rezolvării ecuaţiilor operaţionale prin metode de iteraţie de ordin superior. Lucrările colocviului de teoria aproximării funcţiilor (rezumat), Cluj 15-20 septembrie 1967.
- [7] ††margin: clickable Păvăloiu, I., Sur la méthode de Steffensen pour la résolution des équations opérationnelles non linéaires. Revue Roumaine des Mathématiques Pures et Appliquées, XIII, (1968) 6, pp. 857–861.
- [8] ††margin: clickable Păvăloiu, I., Asupra operatorilor iterativi, Studii şi Cercetări matematice (in print); appeared as: 23 (1971) no. 10, pp. 1567–1574.
- [9] ††margin: clickable Păvăloiu, I., Interpolation dans des espaces linéaires normés et applications. Mathematica, Cluj, vol. 12 (35), 1, 1970, pp. 149–158.
- [10] Stein, M. L., Sufficient conditions for the Banach spaces. Proc. Amer. Math. Soc. 3, pp. 858–863 (1952).
- [11] Traub, J, F., Iterative methods for the solution of equations. Prentice-Hall. Inc. Englewood Cliffs N. J. 1964.
Reçu, 8.VII.1970
